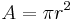Two-dimensional space
Contents |
Details
Bi-dimensional space is a geometric model of the planar projection of the physical universe in which we live. The two dimensions are commonly called length and width. Both directions lie in the same plane.
In physics and mathematics, a sequence of n numbers can be understood as a location in n-dimensional space. When n = 2, the set of all such locations is called 2-dimensional Euclidean space or bi-dimensional Euclidean space.
In physics, our bi-dimensional space is viewed as a planar representation of the space in which we move, described as bi-dimensional space or two-dimensional space.
Two-dimensional geometry
Polytopes
In two dimensions, there are infinitely many regular polytopes: the polygons. The first few are shown below:
Convex
The Schläfli symbol {p} represents a regular p-gon.
| Name | Triangle (2-simplex) |
Square (2-orthoplex) (2-cube) |
Pentagon | Hexagon | Heptagon | Octagon | |
|---|---|---|---|---|---|---|---|
| Schläfli | {3} | {4} | {5} | {6} | {7} | {8} | |
| Image | |||||||
| Name | Nonagon | Decagon | Hendecagon | Dodecagon | Triskaidecagon | Tetradecagon | |
| Schläfli | {9} | {10} | {11} | {12} | {13} | {14} | |
| Image | |||||||
| Name | Pentadecagon | Hexadecagon | Heptadecagon | Octadecagon | Enneadecagon | Icosagon | ...n-gon |
| Schläfli | {15} | {16} | {17} | {18} | {19} | {20} | {n} |
| Image |
Degenerate (spherical)
The regular henagon {1} and regular digon {2} can be considered degenerate regular polygons. They can exist nondegenerately in non-Euclidean spaces like on the surface of a sphere or torus.
| Name | Henagon | Digon |
|---|---|---|
| Schläfli | {1} | {2} |
| Image |
Non-convex
There exist infinitely many non-convex regular polytopes in two dimensions, whose Schläfli symbols consist of rational numbers {n/m}. They are called star polygons and share the same vertex arrangements of the convex regular polygons.
In general, for any natural number n, there are n-pointed non-convex regular polygonal stars with Schläfli symbols {n/m} for all m such that m < n/2 (strictly speaking {n/m}={n/(n-m)}) and m and n are coprime.
| Name | Pentagram | Heptagrams | Octagram | Enneagrams | Decagram | ...n-agrams | ||
|---|---|---|---|---|---|---|---|---|
| Schläfli | {5/2} | {7/2} | {7/3} | {8/3} | {9/2} | {9/4} | {10/3} | {n/m} |
| Image | ||||||||
Hypersphere
The hypersphere in 2 dimensions is a circle, sometimes called a 1-sphere because its surface is one-dimensional. Its area is
where  is the radius.
is the radius.
Coordinate systems in two-dimensional space
The most popular coordinate systems are the Cartesian coordinate system, the polar coordinate system and the geographic coordinate system.
|
|||||||||||||||||